When Should a Team Save its Ace for Game 2?

Photo Credit: Jeff Zelevansky/Icon SMI

Game 1 of a playoff series; matchup of aces, and an electric pitchers’ duel coming up. Whether it's a three-game Wild Card Series, five-game Division Series, or seven-game Championship or World Series, seizing victory in Game 1 and exerting pressure on your opponent is vital.

It seems optimal to have your ace take the mound for that start, granting your team the flexibility to bring him back earlier in the series, and perhaps even from the bullpen in a critical do-or-die scenario. However, can there be exceptions to this conventional philosophy? If Game 1 entails facing off against an elite pitcher such as Randy Johnson, Pedro Martinez, or Jacob DeGrom, could it be wiser to hold your ace for Game 2 to enhance your overall series prospects?

In this analysis, I will explore how a team can alternatively structure its playoff pitching rotation to maximize its chance of winning the series.

The theoretical win % approximations in the tables below are closely related to estimations from Fangraphs’ ZIPS postseason odds and include a homefield advantage factor.*

Wild Card Series

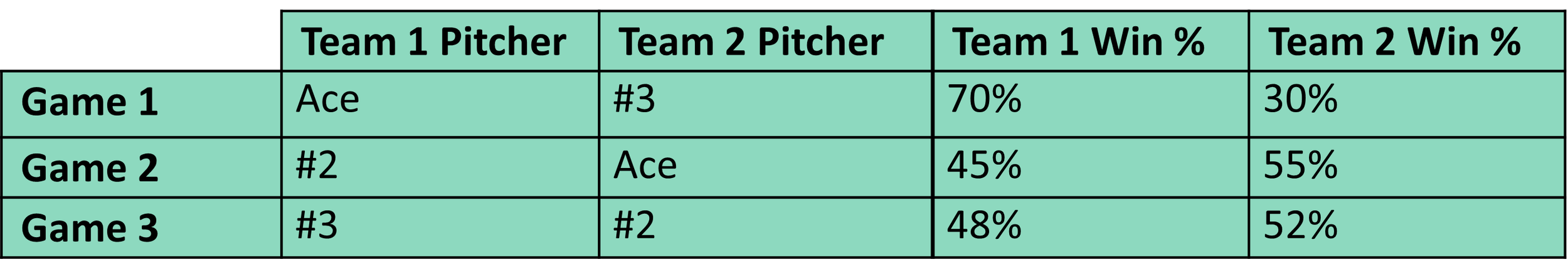
Let’s start by looking at a three-game Wild Card Series.

Table 1: Wildcard Series with traditional pitching matchups

Team 1 is the home team and the overall series favorite in every example. In the example seen in Table 1, Team 1’s Series Win % is 58.48%, calculated by summing the probabilities of them winning Game 1 and Game 2, Game 1 and Game 3, and Game 2 and Game 3.

What if Team 2 pitches its ace in Game 2? Let’s see how this could look like in Table 2.

Table 2: Wildcard Series with alternative pitching matchups

Here, Team 2 lost 10% in win equity in Game 1, but gained 10% in Game 2 and gained 4% in Game 3. Team 1’s Series Win % is now only 56.46%

How would Team 1 counteract this strategy?

Table 3: Wildcard Series with countered pitching matchups

As seen in Table 3, Team 1 is back to 58.48% when it moves its ace to Game 2 as well.

Of the two options that yield Team 2 the same Series Win % based on starting pitcher matchups, is there an option that Team 2 prefers? The latter option reduces the chance that Team 2 gets swept in the series and prevents both aces from coming out of the bullpen in Game 3. Hence, it could be a worthwhile swap for Team 2 to move its ace to Game 2.

How does the probability of a rainout impact this decision making? A rainout may allow both teams to bring back its Game 1 pitcher sooner. So, if an ace vs. ace pitching matchup is unfavorable to your team, forcing your opponent to pitch its ace later in the series is an advantage.

Division Series

Let’s take a look at the two types of five game division series that we’ve seen in the past couple years:

• Type 1: Game 1, Game 2, off day, Game 3, Game 4, off day, Game 5.

• Type 2: Game 1, off day, Game 2, off day, Game 3, Game 4, off day, Game 5.

Switches in homefield advantage come into play here as well, and will be approximated as a 4% shift in win expectancy for each team.

Let’s start by looking at the Type 1 scenario with traditional pitching matchups, shown in Table 4.

Table 4: Division Series Type 1 with traditional pitching matchups

Here, Team 1 is the home team in Games 1, 2, and 5, and Team 2 is the home team in Games 3 and 4. Team 1’s Series Win % is 55.67%.

What if Team 2 pitched its ace in Game 2? Table 5 shows the alternative pitching matchups for this scenario.

Table 5: Division Series Type 1 with alternative pitching matchups

Here, Team 1’s Series Win % is 57.58% when Team 2 moves its ace to Game 2. As seen in Table 5, Team 2’s ace only starts one game in the series after that shift.

As one can expect, Team 2 making that shift and reducing the number of games its ace pitches relative to the number of games its opponent’s ace pitches, is not optimal.

Let’s look at the Type 2 scenario, where there is an extra off day between Game 1 and Game 2:

Table 6: Division Series Type 2 with traditional pitching matchups

Looking at Table 6, we can immediately see that the Type 2 Division Series favors the team with the better #2 pitcher and the team with the worse #4 pitcher, since the #2 pitcher can pitch a second game and the #4 pitcher can be avoided altogether.

Here, Team 1’s Series Win % is 57.89%.

Can Team 2 benefit from pitching its ace in Game 2? Let’s take a look at that in Table 7.

Table 7: Division Series Type 2 with alternative pitching matchups

When Team 2 moves its ace to Game 2, Team 1’s Series Win % drops to 56.42%. From Table 7, we can see that Team 2’s shift made them the favorite in three games, rather than just one game with the traditional matchups.

How will Team 1 counteract this maneuver?

Table 8: Division Series Type 2 with countered pitching matchups

The countered pitching matchups are displayed in Table 8. When Team 1 moves its ace to Game 2, Team 1’s Win % ticks back up to 57.90%.

Hence, it does not seem optimal for a team to pitch its ace in Game 2 of a five game series.

Championship Series or World Series

Finally, let’s consider how an underdog team should match up its pitchers against the favorite with an elite number one pitcher in a seven-game series. Table 9 depicts traditional pitching matchups.

Table 9: Seven-game series with traditional pitching matchups

Team 1 is the home team in Games 1, 2, 6, and 7, and Team 2 is the home team in Games 3, 4, and 5. The seven matchups are stated in Table 9, with Team 1 being the favorite in three of its four home games and in the ace matchup in Game 5.

Here, Team 1’s Series Win % is 56.45%.

What if Team 2 pitched its ace in Game 2?

Table 10: Seven-game series with alternative pitching matchups

When Team 2 moves its ace to Game 2, Team 1’s Series Win % drops to 53.92%. As we can see from Table 10, this switch makes Team 2 the favorite in five of the seven matchups.

How will Team 1 counteract this strategy?

Table 11: Seven-game series with countered pitching matchups

Team 1’s Series Win % increases to 54.83% when it also moves its ace to Game 2. This is still lower than the 56.45% that the traditional strategy from Table 9 yields. Table 11 shows that Team 1 is back to being the favorite in four of the seven games, but its countering maneuver was not able to match its Series Win % with the traditional pitching matchups.

Hence, there are situations in a seven game series in which Team 2 should start its ace in Game 2.

Conclusion

From running simulations with theoretical three-game, five-game, and seven-game series, it does seem like an underdog team, when facing an opponent with a dominant ace, can benefit from starting its ace in Game 2 of a three-game or seven-game series instead of in Game 1. The more dominant the opponent’s ace is, the more the underdog benefits by not matching up its ace against him.

The opponent is likely to adjust and try to match up its ace against the underdog’s ace in Game 2, but that move may not fully offset the advantage gained by the underdog. In addition, forcing the dominant ace to pitch later in the series will reduce his ability to come back in the series for an extra appearance, on short rest, potentially out of the bullpen. Lastly, forcing the dominant ace to pitch later in the series also gives the opponent less of a chance to capitalize on a rain out by bringing the dominant ace back later in the series.


*Flaw of independence: I’m treating game outcomes as independent in this analysis and that is not actually the case. In reality, if an ace pitcher can go deeper into a game and save his team’s bullpen, he can benefit his team the day before and the day after his start.